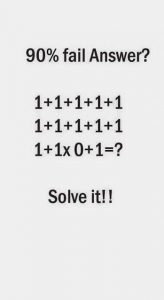Summary: Solutions & Solvers – Mar/Apr/May 19

Mar – May ’19 Brain Teasers – SUMMARY

REMINDERS: Answers in red.  Solvers (submitted/correct) in blue. (Forgive any omissions, but feel free to inform.) Comments in green. For further elaboration, please feel free to ask! 

NOTE:  Solvers of at least one BT:  Frank Green, Kathy Gordon, Rita Barger, Don Hayes, Amy Ragsdale, Jennifer Steele, Alexis Avis, Jim Waterman, and Anita Dixon.

1. In 1982, T.R. Benker set a joke-telling record.  He told jokes continuously for 1803 minutes.  How much longer than one day did he tell jokes? 6 hours, 3 minutes longer than one day. Kathy Gordon, Rita Barger, Amy Ragsdale, Jennifer Steele, Alexis Avis, Jim Waterman.  Partial credit to Frank Green.
2. The widest street in the world is the Monumental Axis in Brasilia, Brazil.  It has 6 lanes and is 0.25 kilometers wide.  If 1 meter = 1.1 yards, how wide is the street in feet?  825 ft. Frank Green, Rita Barger, Amy Ragsdale,  Alexis Avis
3. What is the least common multiple of 54 and 81? 162 How about the greatest common divisor of 16, 41, and 198? 1  Frank, Green, Kathy Gordon, Rita Barger, Amy Ragsdale, Jennifer Steele 
4. (A repeat from May ’17) You have 10 consecutive integers.  The sum of the first 3 is 39.  What is the sum of the last two? 41  Kathy Gordon, Rita Barger, Amy Ragsdale, Jennifer Steele, Alexis Avis
5. Variations on a oft-repeated theme:  a)  Find a proper fraction between 3/7 and 4/9. LOTS(an infinite number) of right answers.  275/630, 43/100, 55/126, . . .  b) Note that if I just added numerators and denominators, I’d get 7/16.  Is 7/16 a solution also?  YES.  Kathy Gordon, Rita Barger, Amy Ragsdale.
6. Of the first 10 counting numbers, three of them have an odd number of factors.  Find the sum of these three numbers. 14 (= 1 + 4 + 9)  Kathy Gordon, Rita Barger, Amy Ragsdale
7. Of the first 25 counting numbers, how many of them have an odd number of factors? 5. (1,4,9,16,25)  Kathy Gordon, Rita Barger, Amy Ragsdale
8. Goldbach’s Conjecture  states (in one equivalent variation) that every even integer greater than 2 can be written as the sum of two primes. Verify this is so for the even integers from 10 to 20. Variety of correct ‘verifications’ here. Kathy Gordon, Rita Barger, Amy Ragsdale       (Bonus Bonus: Prove or disprove this Conjecture in general, and A) win an autographed copy of each of my books, and B) make us both famous! 🙂 ). Sadly, no proofs/disproofs here.  I was hoping we’d be famous! (Lest you wonder:  this is one of the classic unknown problems in what’s called Number Theory.)
9. If the day before yesterday is the 23rd, what is the day after tomorrow?  the 27th.  Kathy Gordon, Rita Barger, Amy Ragsdale
10. See Pic 1 below.  I have one solution for this, but I’m not sure I like it.  Can someone else get others?  GREAT FUN HERE!!  FOUR different answers that work!!  See figure below.  Kathy Gordon, Rita Barger, Amy Ragsdale, Don Hayes, Anita Dixon. (Am I missing someone[s] here? 🙁    Sorry!!)
11. See Pic 2 below (and clarification there).  I’m kinda doubting the ‘90% fail answer’.  If you remember your order of operations, this should be a piece of cake!  12  Kathy Gordon, Rita Barger, Amy Ragsdale
12. Find the largest fraction that a) has a denominator of 17, and b) when added to 1/3, keeps a sum less than 1. 11/17.  Kathy Gordon, Amy Ragsdale, Rita Barger
13. If a certain book is 4th from the left on a bookshelf, and also 6th from the right on the same shelf, how many books are on the shelf?  9.  Kathy Gordon, Amy Ragsdale, Rita Barger
14. (Repeat?) How can you throw a ball as hard as you can and have it come back to you, even if it doesn’t bounce off anything? There is nothing attached to it, and no one else catches or throws it back to you. Throw it straight up. Kathy Gordon, Amy Ragsdale, Rita Barger
15. What is impossibl,e to hold for an hour, even though it hardly weighs anything? Your breath. Kathy Gordon, Rita Barger
16. How many times can you take 3 from 96? Only once.  (After that you’re taking it from 93, 90, etc.) Rita Barger, partial credit to Kathy Gordon.

BONUS #1:  (Ongoing)  What’s the last digit (units place) of the product of the first 100 primes?  0.  (The product of the first 3 primes (2,3,5) is 30.  After that, all future products will retain the 0.)  Rita Barger, Amy Ragsdale.

BONUS #2:  What else do you notice about the numbers you found in #7?  They are perfect squares (among other things).  Kathy Gordon, Amy Ragsdale.

CREATIVITY BONUS #3:  Make as long of a sentence as you can (at least 5 words?) where every word starts with the same letter.  (LOTS of ‘right’ answers, of course!!  Should be fun!)            

Rita Barger’s submission: Arthur, Ann, Alex, Avery, and Arabella are all alert, ambitious, ambidextrous, alarmed, adopted actors actively attracting albatrosses and arresting aardvarks.

Amy Ragsdale’s submission:  Many mischievous monkeys merrily made myriad mango marmalade Monday morning.

BONUS #4:  The grades on six tests are all on a 0 – 100 scale, inclusive.  If the average for the six tests is 93, what’s the lowest possible grade on any one test (in order to maintain the 93 average)? 58.  Kathy Gordon, Rita Barger.

Pic 1:  For #10 above.  Possible Answers: (Are there others?)

1.  Rotate horizontal match on 6 up to become 0.   0 + 4 = 4.
2.  Move vertical match on plus sign to 6, making it 8. 8 – 4 = 4.
3.  Move lower vertical match on 6 (making it 5) to the top of the 4 (making it 9)  5 + 4 = 9.
4.  Move vertical match on plus sign, putting on top of equal sign.  6 – 4 =/ (does not equal) 4.  

Pic 2:  For #11 above.

(NOTE:  Let’s assume there is a + sign at the end of 1st 2 rows.  Thanks to Amy Ragsdale for noticing!)