Jan/Feb ’19 Brain Teasers – SUMMARY
REMINDER: Answers in red. Solvers (submitted/correct) in blue. (Forgive any omissions, but feel free to inform.) Comments in green. For further elaboration, please feel free to ask!
- (An oldie-but-goodie! Too easy?) I have two coins that add up to 55 cents, but one of them is not a nickel. What are the coins? A nickel and half dollar. (One coin is NOT a nickel – the other is.) Frank Green, Kathy Gordon, Rita Barger, Amy Ragsdale, Marcia Morriset, Jennifer Steele.
- How would you divide 55 such that one of the numbers is 1.5 times the other? What are the two numbers? 22 & 33. Frank Green, Kathy Gordon, Rita Barger, Amy Ragsdale, Jennifer Steele.
- I fell off a 30 foot ladder and didn’t get injured. How can this happen? Fell off bottom rung OR ladder was horizontal OR ladder partially submerged in water, among others. Frank Green, Kathy Gordon, Rita Barger, Amy Ragsdale, Marcia Morriset, Jennifer Steele, Dan Felshin.
- The free-throw line on a HS basketball court is 12 feet across, and is a diameter of the half circle which appears above it on the court. The rectangle below the line (going back to the end line) is 12 x 19 feet. (For a more detailed drawing, see FreeThrowLane. ) Together, these two make up the free-throw lane. What is the perimeter of that figure? 6*(PI) + 50 ft. (app 68.8 ft) Frank Green, Amy Ragsdale. (Close: Rita Barger, Kathy Gordon.)
- What is the area of the free-throw lane figure above? 18*(PI) + 228 sq. ft. (app 285.4 sq. ft) Frank Green. (Close: Rita Barger, Kathy Gordon, Amy Ragsdale.)
- Find the 1053rd digit in the decimal expansion of 1/7. 2. Amy Ragsdale.
- A jar is 1/4 full of marbles. If 25 marbles are added to the jar, it becomes one-third full. How many marbles does the jar hold when it’s full? 300 marbles. Rita Barger, Amy Ragsdale.
- A farmer came to town with some melons. He sold half of them plus half a melon, and found that he had one whole melon left. How many melons did he bring to town? 3 melons. Rita Barger.
- (Sound familiar?) Can you put 50 coins into 10 envelopes so that each envelope contains a different number of coins? Depending on conditions, A) impossible, or B) multiple solutions. Credit to Frank Green, Rita Barger, Don Hayes. (Two [or more] recent columns dealt with this problem and a personal anecdote involving Marilyn vos Savant. To (re-)read, see 50Coins1 and/or 50Coins2.)
- A man spends one fifth of the money in his wallet. He then spends one fifth of what remains in the wallet. He spends $36.00 in all. How much money did he have to begin with? $100. Rita Barger, Amy Ragsdale.
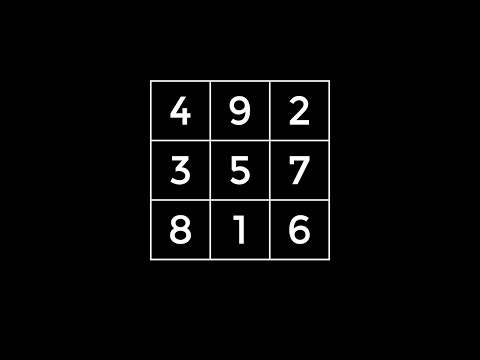
- On a 3 x 3 grid, arrange the digits 1 – 9 (once each) in such a way that each row, each column, and each diagonal adds to 15. (This is called a magic square.) LOTS of answers here. Just one is shown below Problem #12 below. Frank Green, Rita Barger, Amy Ragsdale, Jennifer Steele.
- Jeff Bezos is worth roughly $137 billion. A Mexican peso is worth approximately a nickel. How many pesos is Jeff Bezos worth? (Thanks to subscriber Susie Cook for this one.) 2.74 trillion OR 2 trillion, 740 billion. Frank Green, Amy Ragsdale. (Partial credit to Rita Barger.)
One answer to #11:
BONUS #1: See the figure below. How many squares (of any size) does it contain? (FYI, the link “Click here to see answer” below does not work. 🙂 ) There are 40 squares*. Rita Barger, Amy Ragsdale (Partial credit to Marcia Morriset.)
BONUS #2: (A repeat?) What’s the last digit (units place) of the product of the first 100 primes? Carried over to next set of BTs. Correct solution by Amy Ragsdale.
Figure for Bonus 1:
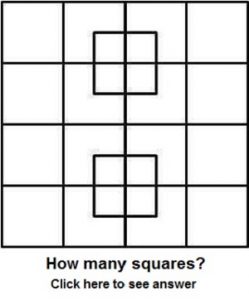
* There are 18 (16 + 2) 1×1 squares, 9 2×2 squares, 4 3×3 squares, 1 4×4 square (the big one), and 8 ‘half unit squares.’ Holler if questions.
